page2
|
COMPACT, a tutorial by Scott W. Williams |
||
|
page 1: |
page 2: |
|
|
page3: |
page4: |
page5: |
2. A SPECIAL EXAMPLE
There are some special compact sets - the convergent
sequence and its limit ![]() ,
the unit interval [0,1],
and the unit circle. These tend to dominate how WE think of compact
objects. The "long" space with an end-point is quite
useful to However, the Cantor set is a fundamentally important
compact object many people believe an aberration - it is not.
Here's one reason why (Urysohn 1914):
,
the unit interval [0,1],
and the unit circle. These tend to dominate how WE think of compact
objects. The "long" space with an end-point is quite
useful to However, the Cantor set is a fundamentally important
compact object many people believe an aberration - it is not.
Here's one reason why (Urysohn 1914):
2.1. THEOREM. Each compact metric space is the continuous image of the Cantor set.
THE CANTOR
(MIDDLE-THIRDS) SET
is the set of all real numbers the limit of an infinite series
of the form ![]() ,
where each is
,
where each is ![]() 0 or
1. Note that if each
0 or
1. Note that if each ![]() =1
(=0), then the sum is 1 (0).
=1
(=0), then the sum is 1 (0).
The construction of the Cantor Middle-Thirds Set proceeds recursively via removing various intervals from [0,1]:
Step1: From
![]()
remove the middle-third open interval (1/3 , 2/3) - we get a closed set, picture
C1:![]()
From both parts of C1 ![]()
remove the middle-third open interval - what's left is a closed set, picture
C2: ![]()
From each of the four parts of
C2: ![]()
remove the middle thirds open interval - picture
C3: ![]()
And continue ...
The Cantor set is the intersection C =![]() Cn.
As an intersection of closed sets in [0,1], C
is compact. As a closed subset of the compact set [0,1], C is closed, and hence, compact.
Cn.
As an intersection of closed sets in [0,1], C
is compact. As a closed subset of the compact set [0,1], C is closed, and hence, compact.
Noticing that the adjacent pairs in the Cantor C set can be mapped in an order preserving manner onto the rationals in (0,1). We see C can be pictured by considering [0,1] and replacing each rational number in (0,1) by two adjacent points. Indeed that picture gives impetus to a special case of Theorem 2.1 - [0,1] is the continuous image of C {just send adjacent points to one}.
Cantor Set
![]()
[0,1]
Concerning the Cantor set, one must be careful with intuition. First it is very thin, because the sum of the lengths of the deleted intervals is 1; i.e., its measure is zero. On the other hand it has the same size as the entire interval [0,1]. This is strengthened by the problem which appears in W. Rudin's textbook, and on some Ph.D. Qualifying Exams:
2.2. EXERCISE. Each real in the interval [0,2] is the sum of two
members of the Cantor set:
For b![]() [0,2]. Consider
the graph Lb
of the intersection of line x+y=b with subsets of the square [0,1]2.
[0,2]. Consider
the graph Lb
of the intersection of line x+y=b with subsets of the square [0,1]2.
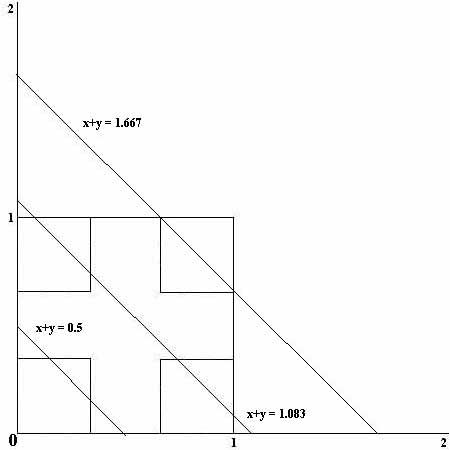
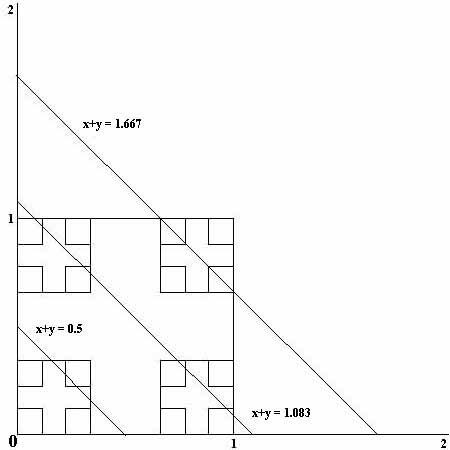
Indeed, each Cn2intersects Lb in a copy of some Ck. Thus,
Lb![]() C2=
C2=![]() (Lb
(Lb![]() Cn2)
is not empty; i.e., there are elements x and y in the Cantor set
such that x+y=b.
Cn2)
is not empty; i.e., there are elements x and y in the Cantor set
such that x+y=b.
In 1999 the great topologist Mary Ellen Rudin solved an outstanding problem generalizing 2.1, which asked for a kind of "triangular inequality" extension of metric. She proved the hard "sufficient" in:
2.3. THEOREM. In order for a compact space X to be the continuous image of a compact linear ordered
space it is necessary and sufficient that for each pair consisting
of a point x![]() X and its neighborhood G, there exists an open set Gx satisfying two conditions:
X and its neighborhood G, there exists an open set Gx satisfying two conditions:
1. x![]() Gx
Gx![]() G.
G.
2. If Gx![]() Hy
Hy
![]() , then either y
, then either y![]() G or x
G or x![]() H.
H.
A pre-print of the paper Nikiel's problem is available at the web site of the journal TOPOLOGY ATLAS (http://at.yorku.ca/topology/)
{Note. The "trianguar inequality" comment is motivated by observing that in a metric space when G is the open ball about x of radius r, we may take Gx to be the open ball about x of radius r/3. Then the triangular inequality proves condition (2)}
![]()
|
page 1: the beginnings |
page 2: a special example |
page3: functional separation |
|
|
page4: the universe in a box |
page5: open covers |
||